Bài giảng: Các bài toán về vectơ - Thầy Nguyễn Công Nguyên

HÌNH 10: 1) Vec tơ 2) Tọa độ mặt phẳng 3) Đường thẳng 4) Đường tròn 5) 3 đường côníc

I. Các khái niệm

1) Định nghĩa

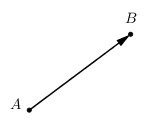
* Vectơ là một đoạn thẳng có hướng. Kí hiệu: \(\overrightarrow {AB} \) * A – gốc; B – ngọn. \(A \equiv B \Rightarrow \overrightarrow 0 \) * AB – độ dài vectơ, \(\left| {\overrightarrow {AB} } \right| = AB\)

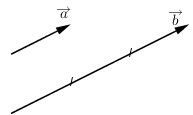
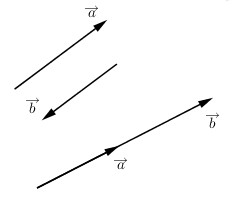
2) Hai vectơ cùng phương: \(\overrightarrow a //\overrightarrow b  \Leftrightarrow \left[ \begin{array}{l}gia\,\,\overrightarrow a //gia\,\,\overrightarrow b \\gia\,\,\overrightarrow a  \equiv gia\,\,\overrightarrow b \end{array} \right.\)

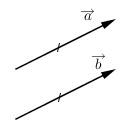
3) Hai vectơ bằng nhau: \(\overrightarrow a  = \overrightarrow b  \Leftrightarrow \left\{ \begin{array}{l}\overrightarrow a  \nearrow  \nearrow \overrightarrow b \\\left| {\overrightarrow a } \right| = \left| {\overrightarrow b } \right|\end{array} \right.\)

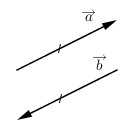
4) Hai vectơ đối: \(\overrightarrow a  =  - \overrightarrow b  \Leftrightarrow \left\{ \begin{array}{l}\overrightarrow a  \nearrow  \swarrow \overrightarrow b \\\left| {\overrightarrow a } \right| = \left| {\overrightarrow b } \right|\end{array} \right.\)

 

III. Các bài toán về vectơ

1) Cộng

* Quy tắc ba điểm: A, B, C bất kì.

 

* Mở rộng: \(\overrightarrow {AB}  + \overrightarrow {BC}  + \overrightarrow {CD}  + \overrightarrow {DE}  = \overrightarrow {AE} \)

* Chú ý: \(\overrightarrow a  + \left( { - \overrightarrow a } \right) = \overrightarrow 0 \)

2) Quy tắc hình bình hành

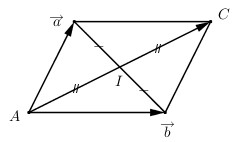
3) Nhận vectơ với số \(k \ne 0\)

\(k\overrightarrow a  = \overrightarrow b \)  khi và chỉ khi: * \(\left| {\overrightarrow b } \right| = k\left| {\overrightarrow a } \right|\) * \(\overrightarrow b \) cùng chiều với \(\overrightarrow a  \Leftrightarrow k > 0\)    \(\overrightarrow b \) ngược chiều với \(\overrightarrow a  \Leftrightarrow k < 0\)

4) Tích vô hướng của 2 vectơ

*  

* \(\overrightarrow a  \bot \overrightarrow b  \Leftrightarrow \overrightarrow a .\overrightarrow b  = 0\)

IV. Các dạng toán về vectơ

1) Dạng 1: Chứng minh hệ thức vectơ

* Phương pháp 1:

* Phương pháp 2: Chứng minh \(\left. \begin{array}{l}VT = P\\VP = P\end{array} \right\} \Rightarrow VT = VP\)

* Công thức:

1. \(\overrightarrow {AB}  + \overrightarrow {BA}  = \overrightarrow 0 \)

2. \(\overrightarrow {AB}  = \overrightarrow {AM}  + \overrightarrow {MB} \)

3. Quy tắc hình bình hành.

V. Bài tập

Bài 1: Cho tứ giác ABCD, M, N là trung điểm AB, CD. Chứng minh \(\overrightarrow {AC}  + \overrightarrow {BD}  = 2\overrightarrow {MN} \) Giải: * Ta có: \(\begin{array}{l}\overrightarrow {AC}  = \overrightarrow {AM}  + \overrightarrow {MN}  + \overrightarrow {NC} \,\,\,\left( 1 \right)\\\overrightarrow {BD}  = \overrightarrow {BM}  + \overrightarrow {MN}  + \overrightarrow {ND} \,\,\,\,\left( 2 \right)\\*\,\,\left( 1 \right) + \left( 2 \right) \Rightarrow \overrightarrow {AC}  + \overrightarrow {BD}  = \left( {\overrightarrow {AM}  + \overrightarrow {BM} } \right) + 2\overrightarrow {MN}  + \left( {\overrightarrow {NC}  + \overrightarrow {ND} } \right)\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \Rightarrow \overrightarrow {AC}  + \overrightarrow {BD}  = \overrightarrow 0  + 2\overrightarrow {MN}  + \overrightarrow 0  = 2\overrightarrow {MN} \,\,\left( {dpcm} \right)\end{array}\)

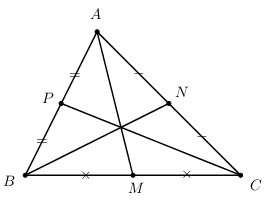
Bài 2: \(\Delta ABC\) có 3 trung tuyến AM, BN, CP. Chưng minh \(\overrightarrow {AM}  + \overrightarrow {BN}  + \overrightarrow {CP}  = \overrightarrow 0 \)

Giải:

* Theo quy tắc hình bình hành có: \(\begin{array}{l}\overrightarrow {AM}  = \frac{1}{2}\left( {\overrightarrow {AB}  + \overrightarrow {AC} } \right)\,\,\left( 1 \right)\\\overrightarrow {BN}  = \frac{1}{2}\left( {\overrightarrow {BA}  + \overrightarrow {BC} } \right)\,\,\,\,\left( 2 \right)\\\overrightarrow {CP}  = \frac{1}{2}\left( {\overrightarrow {CA}  + \overrightarrow {CB} } \right)\,\,\,\,\,\,\left( 3 \right)\\\left( 1 \right) + \left( 2 \right) + \left( 3 \right) \Rightarrow VT = \frac{1}{2}\left[ {\left( {\overrightarrow {AB}  + \overrightarrow {BA} } \right) + \left( {\overrightarrow {AC}  + \overrightarrow {CA} } \right) + \left( {\overrightarrow {BC}  + \overrightarrow {CB} } \right)} \right]\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \frac{1}{2}\left( {\overrightarrow 0  + \overrightarrow 0  + \overrightarrow 0 } \right) = \overrightarrow 0  = VP\end{array}\)

Bài 3: Cho tam giác ABC và điểm M bất kì. G là trọng tâm tam giác ABC. Chứng minh \(\overrightarrow {MA}  + \overrightarrow {MB}  + \overrightarrow {MC}  = 3\overrightarrow {MG} \)

Giải:

* G là trọng tâm tam giác ABC \( \Rightarrow \overrightarrow {GA}  + \overrightarrow {GB}  + \overrightarrow {GC}  = \overrightarrow 0 \)

* Ta có

\[\begin{array}{l}\overrightarrow {MA}  = \overrightarrow {MG}  + \overrightarrow {GA} \,\,\,\left( 1 \right)\\\overrightarrow {MB}  = \overrightarrow {MG}  + \overrightarrow {GB} \,\,\,\left( 2 \right)\\\overrightarrow {MC}  = \overrightarrow {MG}  + \overrightarrow {GC} \,\,\,\left( 3 \right)\\\left( 1 \right) + \left( 2 \right) + \left( 3 \right) \Rightarrow VT = 3\overrightarrow {MG}  + \underbrace {\left( {\overrightarrow {GA}  + \overrightarrow {GB}  + \overrightarrow {GC} } \right)}_{\overrightarrow 0 } = VP\end{array}\]

Bài 4: Cho tam giác ABC và A’B’C’ có trọng tâm lần lượt là G và G’. Chứng minh \(\overrightarrow {AA'}  + \overrightarrow {BB'}  + \overrightarrow {CC'}  = 3\overrightarrow {GG'} \)

Giải:

* Ta có:

\(\begin{array}{l}\overrightarrow {AA'}  = \overrightarrow {AG}  + \overrightarrow {GG'}  + \overrightarrow {G'A'} \,\,\,\,\,\,\left( 1 \right)\\\overrightarrow {BB'}  = \overrightarrow {BG}  + \overrightarrow {GG'}  + \overrightarrow {G'B'} \,\,\,\,\,\,\left( 2 \right)\\\overrightarrow {CC'}  = \overrightarrow {CG}  + \overrightarrow {GG'}  + \overrightarrow {G'C'} \,\,\,\,\,\,\left( 3 \right)\\\left( 1 \right) + \left( 2 \right) + \left( 3 \right) \Rightarrow VT = 3\overrightarrow {GG'}  + \left( {\overrightarrow {G'A'}  + \overrightarrow {G'B'}  + \overrightarrow {G'C'} } \right) - \left( {\overrightarrow {GA}  + \overrightarrow {GB}  + \overrightarrow {GC} } \right)\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 3\overrightarrow {GG'}  + \overrightarrow 0  - \overrightarrow 0  = 3\overrightarrow {GG'} \end{array}\)

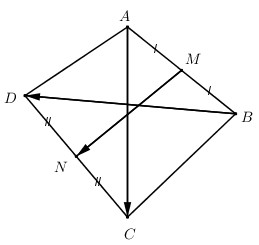
Bài 5: Tứ giác ABCD có M, N là trung điểm của AB, CD. O là trung điểm MN. Chứng minh :

a) \(\overrightarrow {OA}  + \overrightarrow {OB}  + \overrightarrow {OC}  + \overrightarrow {OD}  = \overrightarrow 0 \)

b) I là điểm tùy ý. Chứng minh \(\overrightarrow {IA}  + \overrightarrow {IB}  + \overrightarrow {IC}  + \overrightarrow {ID}  = 4\overrightarrow {IO} \)

Giải:

a) * Ta có: \(\begin{array}{l}\overrightarrow {OA}  + \overrightarrow {OB}  = 2\overrightarrow {OM} \,\,\,\,\left( 1 \right)\\\overrightarrow {OC}  + \overrightarrow {OD}  = 2\overrightarrow {ON} \,\,\,\,\left( 2 \right)\\\left( 1 \right) + \left( 2 \right) \Rightarrow VT = 2\left( {\overrightarrow {OM}  + \overrightarrow {ON} } \right) = \overrightarrow 0  = VP.\end{array}\)  b) * Ta có : \(\begin{array}{l}\overrightarrow {IA}  = \overrightarrow {IO}  + \overrightarrow {OA} \,\,\,\left( 1 \right)\\\overrightarrow {IB}  = \overrightarrow {IO}  + \overrightarrow {OB} \,\,\,\left( 2 \right)\\\overrightarrow {IC}  = \overrightarrow {IO}  + \overrightarrow {OC} \,\,\,\left( 3 \right)\\\overrightarrow {ID}  = \overrightarrow {IO}  + \overrightarrow {OD} \,\,\,\left( 4 \right)\\\left( 1 \right) + \left( 2 \right) + \left( 3 \right) + \left( 4 \right) \Rightarrow VT = 4\overrightarrow {IO}  + \underbrace {\left( {\overrightarrow {OA}  + \overrightarrow {OB}  + \overrightarrow {OC}  + \overrightarrow {OD} } \right)}_{\overrightarrow 0 } = VP\end{array}\)

Bài 6 : Cho tam giác ABC, M, N, P là trung điểm của AB, BC, CA. Chứng minh \(\Delta MNP\) và \(\Delta ABC\) có cùng trọng tâm G.

Học sinh tự giải.

Luyện Bài tập trắc nghiệm môn Toán lớp 10 - Xem ngay